I remember when I learned about the second law of thermodynamics: that the total entropy of a closed system can only increase where entropy is a measure of the disorder of a system.
When I learned this I remember thinking: why did anyone make an equation to describe disorder? How did they make an equation for disorder? And how and why did scientists start to believe, to quote the singer David Byrne from Talking Heads, “things fall apart, it’s scientific”? To answer all of those questions, we need to look to a German man named Rudolf Clausius who had an amazing ability to make almost every other scientist disagree with him for decades before they realized he was fundamentally correct.
Table of Contents
Who is Rudolf Clausius?
How Entropy was discovered
The definition of equivalence-value
Clausius’ Disgregation
The two laws of thermodynamics
References
Who is Rudolf Clausius?

I would like to start in 1849 when Rudolf Clausius was a 27-year-old high school teacher. 1849 was when Clausius read a paper about the theories of heat of a long deceased French scientist named Sadi Carnot. After reading the paper, Clausius decided that some of Carnot’s ideas about heat were correct but he disagreed with the idea that heat is always conserved.
In 1850, Clausius published the theory (that we currently believe is true) that heat can be converted into work and work into heat. Clausius was not the first to promote the idea of heat/work equivalence, but he was the first to state that it does not require one, “to cast the theory of Carnot overboard” but merely the idea that “no heat is lost[1]”.
In addition, Clausius added a new term, which was a combination of what he called the interior work and the interior heat, that we now call the internal energy. With this term, Clausius produced the first complete version of the first law of thermodynamics, that energy is conserved.
Although Clausius didn’t use the word “energy” for another 13 years (as the term “Energy” first became popular in the 1850s and 1860s) an equation that Clausius created is still used to describe the first law with the same letters and sign conventions today! Clausius’s publication made his name in science and soon he was given a job as a professor.
However, it also earned him some enemies. In Scotland, William Thomson(whose paper initially sparked Clausius’s interest)thought that Clausius was just reiterating the work of a disheveled Scottish scientist named William Rankine, and advised Rankine to send a letter to the editor of that effect[2].
In Germany, a scientist named Hermann Helmholtz had just wrote a paper on the conservation of energy, and felt that Clausius was just copying him. In addition, all three men decided that whatever Clausius had not plagiarized was dead wrong. These debates continued for many (many) years and it seems as if every time Clausius published anything he would always get a letter to the editor from Thomson, Rankine, Helmholtz, or more complaining about it.
I tend to side with Clausius on this debate. In fact, as far as I can tell Clausius’s paper of 1850 is startling in it’s accuracy and importance. I’m not alone in this assessment, in 1980, a scientific historian wrote, “There is no doubt that Clausius with this paper (of 1850) created classical thermodynamics…all preceding except Carnot’s is of small moment.
[3]” Also, despite all their objections, Thomson, Rankin, and Helmholtz ended up agreeing with Clausius eventually, although they often retained their distain for the originality of his ideas or the strength of his arguments.
For example, years later Thomson wrote, “The memoir of Clausius contains a most satisfactory and nearly complete working out of the theory of the motive power of heat… [but Clausius’s] hypothesis is so mixed… that the general effect is lost.
[4]” It probably didn’t help that Thomson and Helmholtz were both known for their charm and charisma and Clausius’s personality has been mostly lost to time aside from his brother saying that he was a man of “rare modesty[5]”, his son writing that, “the most principal trait in my father’s character was, without doubt, the splendid truthfulness of his nature” and a letter from a student when Clausius was much older that referred to him as “that old grouch.”
Eventually, both Helmholtz and Thomson were knighted (Helmholtz became Hermann von Helmholtz and Thomson became Lord Kelvin like the temperature scale that is named after him) and Clausius got… um grouchy.
How Entropy was discovered
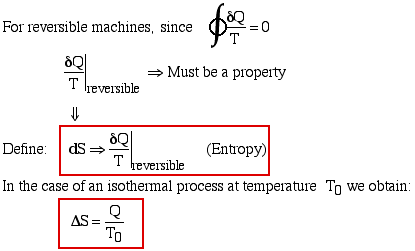
Anyway, despite the attacks, Clausius continued to publish his theories on heat. In 1854, Clausius published his forth paper on heat and this is the one where he created entropy… well sort of.
In this paper, Clausius claimed that in his first paper on heat he had actually stated two laws. First, that heat is a form of energy and energy is conserved, and second, “Carnot’s theorem” that in order for heat to create work, heat must flow from a hot body and to a cold one, and the amount of heat only have to do with the temperature difference.
Clausius felt, however, that “Carnot’s theorem” in “this form…. is incomplete because we cannot recognize therein, with sufficient clearness the real nature of the theorem, and its connection with the first fundamental theorem.[6]” What to do? Clausius knew that Carnot had made this theoretical cycle where if you did the steps in order you used heat to create work but if you did them in reverse you used work to create heat, currently called Carnot’s cycle.
Clausius therefore decided that there had to be some mathematical way to find two heat transformations to be equivalent, so that if you reversed the order, they worked in the opposite way. He declared that this equivalence equation had to be dependent on the heat and some function of the temperature.
He also noted that less heat at lower temperatures was equivalent to more heat at higher temperatures so the temperature must be in the denominator. He then decided that the function he was working on was equal to Q/T where Q is the heat and T is most likely “simply the absolute temperature[7]”.
The definition of equivalence-value

Clausius also defined the “equivalence-value” of heat moving from temperature 1 to 2 as Q(1/T2-1/T1) and gave the letter N for the sum of equivalent values, which he generalized as the integral of the element of heat over Temperature[Note from the present: this function, the heat divided by the absolute temperature is an equation for entropy, although he didn’t call it that yet, now back to Clausius]. Clausius then decided that if a process was reversible, then the sum of these functions must add to zero.
Here is his logic. He then said imagine that it wasn’t true, and the sum was negative. If that was the case then the value of Q(1/T2-1/T1) < 0, which means that heat would have to flow from t2 to t1 or from the lower temperature to the higher temperature, which is not possible due to Carnot’s theorem.
He then added if the sum was positive then you just do the process in reverse and uh oh, you get a negative equivalence-value, which means that heat flows from lower temperatures to higher temperatures, which is again a no-no. Ergo, no matter how complicated a complete cycle is, if it is reversible then the “equivalent values” must add to zero.
Clausius also added that if a process was irreversible, then the sum of the “equivalent values” could not add to a negative number for the same reason as for a reversible cycle but could easily be positive. He therefore wrote “his” second law of thermodynamics to be “the algebraic sum of all transformations occurring in a cyclical process can only be positive.
[8]”By the way, I was taught that heat flows from hotter to colder objects because otherwise it would violate the second law of thermodynamics, but in studying Clausius I have found that the reverse is true, he based his theories on how the “equivalent values” changed on the principle, “Heat cannot by itself pass from a colder to a warmer body.[9]”
Now we fast-forward 8 years to 1862. That was the paper that Clausius looked at his “equivalent values” (cough entropy) equations for all systems, not just ones that went in a full cycle. In this case, an object could reach a new temperature or internal state then it started at.
Clausius’ Disgregation
Clausius decided that the heat usually increases the mean distance between molecules, which he called the “disgregration”, and that an increase in “equivalent values” could be seen in this “disgregration.”
(we no longer use that term, BTW) Clausius noted that water was strange in that when ice melts the molecules actually get closer together so he added that in that case, “the disgregration is not accompanied by [an] increase of the mean distances of its molecules.[10]”
Therefore, Clausius’s “disgregration” just had to do with the orderliness of the molecules. In this paper Clausius became the first to state that one could determine the entropy from the arrangement of molecules inside a body, even if you don’t know how much heat it absorbed!
Also, when Clausius started to look at a single transformation, he realized that, “a general property of… transformations [is that] a negative transformation can never occur without a simultaneous positive transformation whose equivalence-value is at least as great; on the other hand, positive transformations are not necessarily accompanied by negative transformations of equal value, but may take place in conjunction with smaller negative transformations, or even without any at all.
[11]” He concluded, “the algebraic sum of all the transformations occurring during any change of condition whatever can only be positive, or, as an extreme case, equal to nothing.[12]” In other words, entropy of a closed system can only increase. And that is not all.
At the end of this paper, way back in 1862, Clausius concluded with the third law of thermodynamics some 40 years before Walther Nernst, stating that, “it may be proved to be impossible practically to arrive at the absolute zero of temperature by any alteration of the condition of a body[13]”!
The two laws of thermodynamics
Three years later, in 1865, Clausius published his ninth paper about heat, this time he said he was motivated by the desire to, “bring the second fundamental theorem, which is much more difficult to understand that the first, to its simplest and at the same time most general form.
[14]” In this paper, Clausius decided to give the expression of heat over temperature the letter S for no reason I can tell and also decided to rename it from the “equivalence value” to a new shorter term that he made up called “entropy”. We still use the letter S for entropy because of Clausius.
In 1865, Clausius wrote that the word “entropy” comes from the Greek word for transformation and he “intentionally formed the word entropy so as to be as similar as possible to the word energy.
[15]” Clausius then concluded with his version of the two laws of thermodynamics: “1. The energy of the universe is constant. 2. The entropy of the universe tends to a maximum.[16]”Clausius’s version of the first two laws in 1865 is still considered correct today!
Meanwhile, William Thomson, one of Claudius’s big critics, was creating his own “second law” albeit without a corresponding equation. In 1852, Thomson wrote that there was always a, “waste of mechanical energy available to man when heat is allowed to pass from one body to another at a lower temperature.
[17]” By 1862, Thomson decided that, “the second great law of thermodynamics involves a certain principle of irreversible action in Nature. It is thus shown that, although mechanical energy is indestructible, there is a universal tendency to its dissipation, which produces a gradual augmentation and diffusion of heat, cessation of motion, and exhaustion of potential energy through the material universe.
[18]“ In 1865, when Clausius named his function entropy, Clausius also realized that his equations and theories of entropy worked perfectly with Thomson’s ideas of “wasting energy”! Suddenly the irreversible action of nature had a corresponding equation.
But wait you say (or you might say), heat over temperature is not the definition of entropy I learned in school! That is probably because you (and I) were taught Boltzmann’s entropy equation S = klogW.
You won’t be surprised to learn from the name that Clausius did not create Boltzmann’s entropy formula. But you might be surprised to learn that Boltzmann didn’t create it either, even though it is carved onto his gravestone. So how did we get from Clausius to Boltzmann’s equation and why are his equation and constant named after him if he didn’t directly create them? That is next time on the lightning tamers.
References
[1]“to cast the theory of Carnot overboard…”Clausius, R “First Memoir” (1850) The Mechanical Theory of Heat (1867) p. 17
[2] In August, 1850 Rankine wrote to William Thomson thanking him for “calling my attention to the paper by Clausius … I approve of your suggestion to send a copy of my paper either to Clausius or Poggendorff” Found in Smith, Crosbie Energy and Empire: A Biographical Study of Lord Kelvin (1989) p. 320
[3]“There is no doubt that Clausius…”Truesdell, C The Tragicomical History of Thermodynamics (2013)p. 204
[4]“…[Clausius’s] hypothesis is so mixed…” Found in Smith, Crosbie Energy and Empire: A Biographical Study of Lord Kelvin (1989) p. 343
[5]“rare modesty“ Robert Clausius “Obituary Notices of Fellows Deceased” Proc. Royal Society of LondonVol. 48 (Dec 31, 1891) p. 292-3
[6]“this form…. is incomplete…”Clausius, R “Fourth Memoir” (1854) The Mechanical Theory of Heat (1867) p. 111
[7]“simply the absolute temperature…” Clausius, R “Fourth Memoir” (1854) The Mechanical Theory of Heat (1867) p. 135
[8]“the algebraic sum of all…”Clausius, R “Fourth Memoir” (1854) The Mechanical Theory of Heat (1867) p. 133
[9]“Heat cannot by itself…” Clausius, R “Fourth Memoir” (1854) The Mechanical Theory of Heat (1867) p. 117
[10]“the disgregration is not accompanied …” Clausius, R “Sixth Memoir” (1862) The Mechanical Theory of Heat (1867) p. 222
[11]“a general property of… transformations …” Clausius, R “Sixth Memoir” (1862) The Mechanical Theory of Heat (1867) p. 244
[12]“the algebraic sum of all the transformations…”Clausius, R “Sixth Memoir” (1862) The Mechanical Theory of Heat (1867) p. 247
[13]“it may be proved to be impossible practically to arrive at the absolute zero…” Clausius, R “Sixth Memoir” (1862) The Mechanical Theory of Heat (1867) p. 250
[14]“bring the second fundamental theorem…” Clausius, R “Ninth Memoir” (1865) The Mechanical Theory of Heat (1867) p. 327
[15]“intentionally formed the word entropy…”Clausius, R “Ninth Memoir” (1865) The Mechanical Theory of Heat (1867) p. 357
[16]“1. The energy of the universe is constant. 2. The entropy …” Clausius, R “Ninth Memoir” (1865) The Mechanical Theory of Heat (1867) p. 365
[17]“waste of mechanical energy available to man …” Thomson, W “On a Universal Tendency in Nature to the Dissipation of Mechanical Energy” The Philosophical Magazine Vol. 4 No. iv (1852) p. 304
[18]“the second great law of thermodynamics…”Thomson, W “On the Age of the Sun’s Heat” Macmillan’s Magazinevo. 5 (March 5, 1862). P. 388
/*54745756836*/